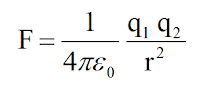Saturday, February 18, 2017
Tuesday, February 14, 2017
Friday, February 10, 2017
CHAPTER (9)
ELECTRIC POTENTIAL
လွ်ပ္စစ္အတည္စြမ္းအင္း (Voltage)
Work and potential energy have already been studied in mechanics. The concepts of work and potential energy are also very useful in the study of electrical phenomena. Let us review What we have learned thus far about these concepts.အလုပ္ (Work) ႏွင့္ အတည္စြမ္းအင္း (potential energy) တို႔ကို mechanics သင္ခန္းစာမ်ားတြင္ ေလ့လာထားၾကၿပီးျဖစ္သည္။ ၎ work and potential energy တို႔၏ပညာရပ္မ်ားသည္ လွ်ပ္စစ္အလုပ္အေဘာတရား၏ပညာရပ္တြင္ အလြန္အသံုး၀င္ျပန္ပါသည္။ ၎ ပညာရပ္မ်ားႏ်ွပ္ပတ္သတ္ေသာ ကြ်န္ေတာ္တို႔ေလ့လာထားခ်က္မ်ားကို ျပန္လွန္ေလ့လားၾကည့္ၾကပါစို႕။
In Fig. 9.1(a), a body of mass m is situated on the ground. When the body is lifted to a certain height, work is done against the gravitational force mg. If the body is lifted to a height h, the work done is mgh [Fig. 9.1 (b)]. This work does not disappear but resides in the body as potential energy. This means that the body has potential energy with respect to the ground. So, external work must be done to separate two bodies which attract each other. The work done is then transformed into the potential energy of the body.
အထက္ပါပံု Fig. 9.1 (a) တြင္ ျဒပ္ထုအေလးခ်ိန္ m ရွိေသာ အရာ၀တၳဳတစ္ခုသည္ ေျမျပင္ေပၚတြင္တည္ရွိသည္။ ၎ အရာ၀တၳဳကို အျမင့္ h ေနရာသို႔မွတင္လိုက္ေသာအခါ ကမၻာေျမဆဲြအား (mg) ကိုတြန္းကန္လွ်က္ Work တစ္ခုကိုစိုက္ထုတ္ရပါမည္။ အထက္ပါပံု (b)တြင္ ၎ အရာ၀တၳဳကို အျမင့္ h ေနရာသို႔မွတင္လိုက္လွ်င္ Work done သည္ mgh ျဖစ္မည္။ ၎ အရာ၀တၳဳတြင္ work သည္ ပ်က္ျပယ္မသြားေသာ္လည္း potential energy သည္ ျပန္၍တည္ရွိျဖစ္ေပၚလာသည္။ ဆိုလိုသည္မွာ ေျမျပင္ႏွင့္သက္ဆိုင္ေသာ potential energy ကို ၎ အရာ၀တၳဳသည္ ပိုင္ဆိုင္ျဖစ္ေပၚလာသည္။ ဤကဲ့သိုမွတင္ရာတြင္ တစ္ခုႏွင့္တစ္ခုကို ဆဲြငင္းေနၾကေသာ အရာႏွစ္ခု (body and ground) ကို ကြာေ၀းကဲြျခားေစရန္ ျပင္ပ work (external work) တစ္ခုကို စိုက္ထုတ္ရမည္။ external work ကိုစိုက္ထုတ္ရင္းျဖင့္ work done သည္ potential energy အျဖစ္သို႔ကူးေျပာင္းသြားသည္။
If the body is released it will fall to the ground [Fig.9.1 (c)]. While falling to the ground its potential energy gets less and less. But as it falls, the speed increases and therefore its kinetic energy also increases. The potential energy of the body is changing gradually into kinetic energy. As soon as the body strikes the ground the potential energy is totally transformed into kinetic energy. It has been described in mechanics that the kinetic energy of the body when it strikes the ground is equal to the work done in lifting the body to the height h. Thus as soon as the body strikes the ground the potential energy store while it is at the height is completely converted into kinetic energy. In other words, work is done on the body falling from a height by the gravitational field.
အထက္ပါပုံ (c) အရ ၎အရာ၀တၳဳကို လြတ္ခ်လိုက္လွ်င္ ၎အရာ၀တၳဳသည္ ေျမျပင္ကိုက်ေရာက္သြားလိမ္မည္။ ေျမျပင္သိုလြတ္က်ေနစဥ္တြင္ ၎အရာ၀တၳဳ၏ potential energy သည္ နည္းသထက္နည္းသြားလိမ္မည္။ သို႕ေသာ္လည္း ၎အရာ၀တၳဳသည္လြတ္က်ေနစဥ္တြင္ speed သည္ တုိးလာေသာေၾကာင့္ kinetic energy (KE) သည္လည္း တိုးလာလိမ္မည္။ ၎အရာ၀တၳဳ၏ potential energy သည္ kinetic energy အျဖစ္သို႔ တစ္ျဖည္းျဖည္းခ်င္းေျပာင္းလည္းေနမည္။ ၎အရာ၀တၳဳသည္ ေျမျပင္သိုရုိက္ခတ္ခ်ိန္တြင္ potential energy သည္ kinetic energy သို႔ လုံး၀ေျပာင္းလဲသြားသည္။ အရာ၀တၳဳတစ္ခုသည္ ေျမျပင္သို႔ရိုက္ခတ္ေသာအခ်ိန္ Kinetic Energy (အေရြ႕စြမ္းအင္) သည္ ၎အရာ၀တၳဳကို အျမင့္ (h) တစ္ေနရာဆီသို႔ျမင့္တင္ထားေသာအခ်ိန္တြင္ ျပီးေျမာက္ေသာ work done ပမာဏႏွင့္တူညီသည္ကို mechanic သင္ခနး္စာမ်ားတြင္ ေဖာ္ျပထားခဲ့ၾကၿပီးျဖစ္သည္။ ဤနည္းအားျဖင့္ ေျမျပင္သို႔ရိုက္ခတ္ခ်ိန္တြင္ အျမင့္ တစ္ေနရာအတြင္သိုေလွာျဖစ္ေပၚေနသာ Potential Energy သည္ Kinetic Energy အျဖစ္သို႔လံုး၀ေျပာင္းလဲသြားသည္။ တစ္နည္းအားျဖင့္ဆိုေသာ ကမၻာစက္ကြင္းဆဲြအားေၾကာင့္ အျမင့္တစ္ေနရာမွ ျပဳတ္က်လာေသာ အရာ၀တၳဳေပၚတြင္ Work တစ္ခုကို စိုက္ထုတ္လုပ္ေဆာင္ျပီးစီးသြားသည္ျဖစ္သည္။
Although the above facts concern the gravitational force, they are also true for electric forces. This means that work must be done to separate two bodes having opposite charges since they attract each other. Likewise, work must be done to bring closer two bodies having the same kind of charge since they repel each other. In both cases the work done is stored up as electric potential energy in the charged bodies.
အထက္ပါေဖာျပခ်က္မ်ားသည္ gravitational force ႏွင့္သက္ဆိုင္ေသာအခ်က္မ်ားျဖစ္ေသာ္လည္း ၎အခ်က္မ်ားသည္ electric forces မ်ားတြင္လည္း မွန္ကန္ေစပါသည္။ ဆိုလိုသည္မွာ တစ္ခုကိုတစ္ခုဆဲြငင္ေနၾကေသာ opposite charges မ်ားရွိသာ အရာ၀တၳဳႏွစ္ခုကို ခဲြျခားရန္အတြက္ work ကို စိုက္ထုတ္ရမည္ျဖစ္သည္။ တစ္နည္းဆိုေသာ္ တစ္ခုကိုတစ္ခုတြန္းကန္ေနၾကေသာ အမ်ဳိးတူ charge မ်ားပိုင္ဆိုင္ေသာ အရာ၀တၳဳမ်ားကို နီးကပ္ေအာင္ယူေဆာင္ျခင္းတြင္ work ကို စိုက္ထုတ္လုပ္ေဆာင္ေစရမည္ျဖစ္သည္။ ထိုအခ်က္ႏွစ္ခ်က္တြင္ လွ်ပ္စစ္ဓါတ္ေဆာင္ အရာ၀တၳဳမ်ားတြင္ work done သည္ potential energy ျဖစ္သို႔သိုေလွာင္လုပ္ေဆာင္သြားသည္။
ELECTRIC POTENTIAL AND POTENTIAL DIFFERENCE
The electric filed around the charge +Q, Shown in Fig. 9.2, will now be considered. It has been found that the direction of the electric filed around +Q is radially outward. The points A and B are in the electric filed around +Q.ယခုအခါ ပံု (Fig, 9.2) တြင္ ျပထားေသာ charge +Q ၏ပတ္၀န္းက်င္တြင္ရွိေသာ electric field ကိုဆက္ဆက္ေတြးေခၚၾကမည္။ Charge +Q ပတ္၀န္းက်င္တြင္ရွိေသာ electric field ၏ direction သည္ ဗဟုိျပဳခ်က္တည္လွ်က္ အျပင္သို႔ဦးတည္သြားသည္ကိုေတြ႕ရွိျပီးသားျဖစ္သည္။ အမွတ္ A ႏွင့္ အမွတ္ B တို႔သည္ Charge +Q ပတ္၀န္းက်င္တြင္ျဖစ္ေပၚေသာ electric field ထဲမွက်ေရာက္ေနေသာ အမွတ္မ်ားျဖစ္ၾကသည္။
Fig. 9.2 Electric potential at a point in an electric field
When a small positive charge q is placed at A the charges Q and q repel each other. The repulsive force acting on q is F = qE. When q is brought to B, a point which is closer to Q, work must be done against the electric force. This work has been transformed into electric potential energy of q at B. This means that the small positive charge q has gained potential energy.
ေသးငယ္ေသာ positive charge q ကို အမွတ္ A ေနရာ၌ထားေသာအခါ ၎ charge Q ႏွင့္ q တို႔သည္ တစ္ခုကိုတစ္ခု တြန္းကန္ၾကသည္။ Charge q ေပၚသုိ႕သက္ေရာက္ေနေသာ repulsive force (တြန္းအား) သည္ F = qE ျဖစ္သည္။ charge q ကို charge Q ႏွင့္ပိုိနီးေသာ point B သို႔ယူသြားေသာအခါ electric force ကို ခုခံလွ်က္ work ကို စိုက္ထုတ္ေစရပါမည္။ ထိုသို႔စုိက္ထုတ္လိုက္ေသာ work သည္ အမွတ္ B ၌ ရွိေသာ charge q ၏ electric potential energy အျဖစ္သုိ႔ေျပာင္းလဲးသြားသည္။ ဆိုလိုသည္မွာ ၎ေသးငယ္ေသာ positive charge q သည္ potential energy ကိုရရွိသြားသည္။
Let us suppose that q is initially not at A but at infinity. If q is now brought to B work must again be done and hence q gains electric potential energy. If instead of q a unit positive charge is brought from infinity to B, then the unit positive charge will gain electric potential energy. The electric potential energy of the unit positive charge at B is defined as the electric potential at B. The electric potential may therefore be defined as follows.
charge ကို အမွတ္ A တြင္ မရွိပဲ infinity အေ၀းတစ္ေနရာတြင္ရွိသည္ဟု ယူဆၾကပါစို႔။ ယခုအခါ charge q ကို အမွတ္ B သို႔ ယူေဆာင္လွ်င္ ထပ္မံျပန္တစ္ခါ work ကို စိုက္ထုတ္လုပ္ေဆာင္ရမည္။ ထိုေၾကာင့္ပင္ charge q သည္ electric potential energy ကုိ ထပ္မံရရွိျဖစ္ေပၚမည္။ charge q အစား အျခားေသာ တစ္ယူနစ္ positive charge တစ္ခုကို infinity မွ အမွတ္ B ဆီသို႔ သယ္ေဆာင္လွ်င္ ၎ positive charge သည္လည္း ထပ္မံ၍ electric potential energy ကိုရရွိေနဦးမည္ျဖစ္သည္။ အမွတ္ B ၌ရွိေသာ ၎ positive charge ၏ electric potential energy ကို အမွတ္ B ၌ရွိေသာ electric potential အျဖစ္သတ္မွတ္ယူသည္။ ထို႕ေၾကာင့္ပင္ electric potential ကို ောက္ပါအတိုင္းဖြင့္ဆိုႏိုင္သည္။
The electric potential at a point in an electric filed is the work done in bringing a unit positive charge against the electric force from infinity to that point.
လွ်ပ္စစ္စက္ကြင္းအတြင္ အမွတ္တစ္ေနရာ၌ရွိေသာ electric potential ဆိုသည္မွာ infinity အေ၀းတစ္ေနရာမွ ထိုအမွတ္ဆီသို႔ electric force ကိုခုခံလွ်က္ အေပါင္းတစ္ယူနစ္ charge ကို သယ္ေဆာင္ျခင္းငွာ ျပီးေျမာက္ေသာ work done ကိုေခၚသည္။
Let W be the work done in bringing the small positive charge q from infinity to a point in the electric field around Q. If V is the electric potential at that point, Then it may be expresses as
W ကို charge Q ပတ္၀န္းက်င္တြင္ရွိေသာ electric field အတြင္းရွိ infinity အေ၀းတစ္ေနရာမွ အမွတ္တစ္ခုဆီသို႔ positive charge q ကို သယ္ေဆာင္ျခင္းတြင ၿပီးေျမာက္ေသာ work done အျဖစ္ယူဆထားပါ။ V သည္ ထုိအမွတ္၌ရွိေသာ electric potential ျဖစ္လွ်င္ ေအာက္ပါအတိုင္း equation ေဖာ္ျပႏိုင္သည္။
electric potential သည္ work done ၏ ပမာဏျဖစ္ေသာေၾကာင့္ electric potential သည္ scalar quantity အမ်ိဳးအစားျဖစ္သည္။ အျပည္ျပည္ဆိုင္ရာအဖဲြ႕အစည္းသည္ infinity အေ၀းတစ္ေနရာ၌ရွိေသာ electric potential ကို Zero သုည အျဖစ္မွတ္ယူသည္။ charge Q ပတ္၀န္းက်င္ရွိ electric field အတြင္းရွိ အမွတ္တစ္ေနရာ၌ရွိေသာ electric potential ကို infinity ၌ရွိေသာ electric potential ႏွင့္ relative ( မ်ဳိးတူ ) အျဖစ္ ေဖာ္ျပၾကသည္။
In Fig. 9.2 if the unit positive charge brought to B is set free it will move away from Q to infinity. While moving away from Q its electric potential decreases gradually and becomes zero when it is back at infinity. The electric force does work on the unit positive charge while it is moving away from Q.
positive charge ကို အမွတ္ B သို႔ယူေဆာင္သြားလွ်င္ ၎ positive charge သည္ charge Q မွ infinity အေ၀းသို႔ လြတ္လပ္စြာ ေရြ႕သြားလိမ္မည္ (+Q ႏွင့္ +q မ်ဳိးတူ charge ေတြတြန္းကန္မူေၾကာင့္)။ ထို႕ကဲ့သို charge q သည္ charge Q မွ အေ၀းသို႔ေရြ႕လွ်ားေနစဥ္ ၎ charge q ၏ electric potential သည္ တစ္ျဖည္းျဖည္း နည္းသထက္နည္းလားျပီး infinity သို႔ျပန္ေရာက္ခ်ိန္တြင္ charge q ၏ electric potential သည္ Zero ျဖစ္သြားသည္။ ၎ positive charge သည္ charge Q မွအေ၀းသိုေရြ႕လွ်ားေနစဥ္အတြင္း electric force သည္ ၎ positive charge q အေပၚတြင္ work တစ္ခုကိုလုပ္ေဆာင္ေနသည္။
The Unit of Electric Potential
The practical unit of electric potential is volt (V ). If the work done in bringing +1 coulomb from infinity to a point in an electric filed is 1 Joule, the electric potential at that point is 1 joule per coulomb (1 J C⁻¹ ) or 1 V.electric potential ၏ လက္ေတြ႔သံုး unit သည္ V ျဖစ္သည္။ electric field တစ္ခုအတြင္း infinity အေ၀းတစ္ေနရာမွ အမွတ္တစ္ခုဆီသို႔ charge ပမာဏ +1 coulomb ကို သယ္ေဆာင္ခ်ိန္တြင္ ၿပီးေျမာက္ေသာ work done သည္ 1 Joule ရွိွလွ်င္ ထိုအမွတ္၌ရွိေသာ electric potential တန္ဖိုးသည္ 1 joule per coulomb (1 J C⁻¹ ) or 1 V ရွိသည္။
The Electric Potential Difference
In Fig. 9.3 the points A and B are in the electric field of a point charge +Q. A is at a distance of "a" from +Q and B is at a distance of "b" from +Q. Then, the distance between A and B is ( a - b ).
Fig. 9.3 The electric potential difference between two points in an electric field
႔ပံု Fig 9.3 တြင္ အမွတ္ A ႏွင့္ အမွတ္ B တို႔သည္ အမွတ္ charge Q ၏ electric field အတြင္းတြင္ရွိၾကသည္။ အမွတ္ A သည္ charge +Q မွ အကြာေ၀း "a" ၌ရိွေသာအမွတ္ျဖစ္ၿပီး၊ အမွတ္ B သည္ charge +Q မွ အကြာေ၀း "b" ၌ရိွေသာအမွတ္ျဖစ္သည္။ အမွတ္ A ႏွင့္ အမွတ္ B ၾကားတြင္ရွိေသာ အကြားအေ၀းမွာ ( a - b ) ျဖစ္သည္။
Let VA be the electric potential at A and VB be the electric potential at B. By definition, VA and VB can be expressed as follows.
အမွတ္ A ၌ ရွိေသာ electric potential သည္ VA ျဖစ္ယူဆထားျပီး၊ အမွတ္ B ၌ ရွိေသာ electric potential သည္ VB ျဖစ္ယူဆထားပါ။ ေဖာ္ျပခ်က္အားျဖင့္ VA ႏွင့္ VB တို႔ကုိေအာက္ပါအတိုင္းေဖာ္ျပႏိုင္သည္။
VA = the work done in bringing a unit positive charge from infinity to A.
VB = the work done in bringing a unit positive charge from infinity to B.
= the work done in bringing a unit positive charge from infinity to A + the work done
in bringing a unit positive charge from A to B.
= VA + the work done in bringing a unit positive charge from A to B
VA သည္ (အေ၀းတစ္ေနရာ infinity မွ အမွတ္ A ေနရာသို႔ တစ္ယူနစ္ positive charge ကို သယ္ေဆာင္ျခင္းငွာ ျပီးေျမာက္ေသာ work done) ႏွင့္ညီသည္။
VB သည္ (အေ၀းတစ္ေနရာ infinity မွ အမွတ္ B ေနရာသို႔ တစ္ယူနစ္ positive charge ကို သယ္ေဆာင္ျခင္းငွာ ျပီးေျမာက္ေသာ work done) ႏွင့္ညီသည္။
VB သည္ (အေ၀းတစ္ေနရာ infinity မွ အမွတ္ A ေနရာသို႔ တစ္ယူနစ္ positive charge ကို သယ္ေဆာင္ျခင္းငွာ ျပီးေျမာက္ေသာ work done) ႏွင့္ (အမွတ္ A မွ အမွတ္ B ေနရာသို႔ တစ္ယူနစ္ positive charge ကို သယ္ေဆာင္ျခင္းငွာ ျပီးေျမာက္ေသာ work done) တို႔ ေပါင္းျခင္းႏွင့္တူညီသည္။
VB သည္ (အမွတ္ A ၌ရွိေသာ electric potential) ႏွင့္ (အမွတ္ A မွ အမွတ္ B ေနရာသို႔ တစ္ယူနစ္ positive charge ကို သယ္ေဆာင္ျခင္းငွာ ျပီးေျမာက္ေသာ work done) တို႔ ေပါင္းျခင္းႏွင့္တူညီသည္။
Therefore,
VB - VA = the work done in bringing a unit positive charge from A to B.
ထို႔ေၾကာင့္ VB - VA သည္ အမွတ္ A မွ အမွတ္ B သို႔ တစ္ယူနစ္ positive ကို သယ္ေဆာင္ျခင္းငွာ ျပီးေျမာက္ေသာ work done ႏွင့္ ညီသည္။
But VB - VA is the electric potential difference between A and B. The electric potential difference between two points in an electric field can be defined as follows.
သို႔ေသာ္လည္း VB - VA သည္ အမွတ္ A ႏွင့္ အမွတ္ B ၾကားတြင္ရွိေသာ electric potential difference သာလွ်င္ျဖစ္သည္။ electric field တစ္ခုအတြင္း အမွတ္ႏွစ္ခုၾကားတြက္ရွိေသာ electric potential difference ေအာက္ပါအတိုင္းဖြင့္ဆိုႏိုင္သည္။
The electric potential difference between two points in an electric field is the work done in bringing a unit positive charge from one point to another against electric forces.
လွ်ပ္စစ္စက္ကြင္းတစ္ခုအတြင္း အမွတ္ႏွစ္ခုၾကားတြင္ရိွေသာ electric potential difference ဆိုသည္မွာ electric forces ကိုခုခံေက်ာ္လြားလွ်က္ အမွတ္တစ္ေနရာမွ အျခားအမွတ္တစ္ေနရာသို႔ တစ္ယူနစ္ positive charge ကိုသယ္ေဆာင္ျခင္းငွာ ျပီးေျမာက္ေသာ work done ပမာဏကိုဆိုလိုသည္။
The Unit of Electric Potential Difference
If the work done in bringing a charge of +1C from one point to another in an electric field is 1 J, the electric potential difference difference between those points is 1 V.
electric field တစ္ခုအတြင္း အမွတ္တစ္ခုမွ အျခားအမွတ္တစ္ခုသို႔ +1C ရွိေသာ charge တစ္ခုကို သယ္ေဆာင္ျခင္းတြင္ ျပီးေျမာက္ေသာအလုပ္သည္ 1 J ရွိလွ်င္ ၎အမွတ္ႏွစ္ခုၾကားတြင္ရွိေသာ electric potential difference မွာ 1 V ရိသည္။
In Fig. 9.3 the electric potential at B is higher than that at A. If a small positive charge is placed at B it will move toward A since it is repelled by +Q. A small positive charge will move from a point of higher electric potential to a point of lower electric potential. If a small negative charge is placed at A it will move toward B since it is attracted by +Q. A small negative charge will move from a point of lower electric potential to a point of higher electric potential.
ပံု Fig. 9.3 တြင္ အမွတ္ B ၌ရွိေသာ electric potential သည္ အမွတ္ A ၌ရိွေသာ electric potential ထက္ ၾကီးမားျမင့္မားသည္။ positive charge တစ္ခုကို အမွတ္ B သို႔သြားထားလွ်င္ ၎ positive charge သည္ charge +Q ၏ တြန္းကန္ျခင္းေၾကာင့္ အမွတ္ A ေနရာဆီသိုေရြ႕သြားမည္။ ၎ positive charge သည္ electric potential ျမင့္ေသာအမွတ္ေနရာမွ electric potential ႏွိမ့္ေသာ အမွတ္ေနရာဆီသို႔ ေရြ႕သြားလိမ္မည္။ Negative Charge ကို အမွတ္ A ေနရာ၌ထားလွ်င္ ၎ negative charge သည္ charge +Q ၏ဆဲြအားေၾကာင့္ အမွတ္ B ေနရာသို႔ေရြ႕သြားမည္ျဖစ္သည္။ ၎ negative သည္ electric potential ႏွိမ့္ေသာေနရာမွ electric potential ျမင့္ေသာေနရာဆီသို႔ ေရြ႕သြားလိမ့္္မည္ျဖစ္သည္။
The Electric Potential due to a Point Charge
The electric potential at a distance r from a point charge +Q can be expressed ascharge Q ေနရာမွ အကြာအေ၀း r ၌ရွိေသာ electric potential ကို ေအာက္ပါအတိုင္းေဖာ္ျပႏိုင္သည္။
ထို႔ေၾကာင့္ အမွတ္တစ္ေနရာ၌ရွိေသာ electric potential V သည္ charge Q ကို တိုက္ရိုက္အခ်ိဳးက်ေနျပီး၊ ၎အမွတ္ႏွင့္ charge Q ၾကား အကြာေ၀းကို ေျပာင္းျပန္အခ်ဳးိက်သည္။
Suppose that the total electric potential at a point due to several point charges is to be determined. First, the electric potentials at that point due to the individual charges must be calculated. In doing so the signs of individual charges must be taken into account. That is to say the individual electric potentials must be added algebraically. If the electric potentials due to the charges +Q₁, + Q₂, + Q₃, ............, are V₁, V₂, V₃, ................., respectively, the total electric potential V is
V = V₁ + V₂ + V₃ + .........................
မ်ားစြာေသာ point charge မ်ားေၾကာင့္ အမွတ္တစ္ေနရာ၌ရွိေသာ စုစုေပါင္း electric potential ကို တြက္ယူဆံုးျဖတ္ရန္ရွိသည္ဟုမွတ္ယူထားပါ။ ပထမဦးစြာ charge တစ္ခုခ်င္းစီေၾကာင့္ ထို႔အမွတ္ေပၚ၌သက္ေရာက္ေနၾကေသာ electric potential ကို တြက္ယူရမည္ျဖစ္သည္။ ဤလုပ္ေဆာင္တြက္ယူျခင္းတြင္ charge တစ္ခုခ်င္းစိ၏ လကၡဏ (+ or -) ကို တြက္ယူျခင္းတြင္ ယူေဆာင္တြက္ခ်က္ရမည္။ ဆိုလိုခ်င္သည္မွာ တစ္ခုခ်င္းစီေသာ electric potential မ်ားကို သခ်ာၤကိန္းမ်ားအားျဖင့္ ေပါင္းတြက္ရမည္ျဖစ္သည္။ charges +Q₁, + Q₂, ,+ Q₃ ............
စသည္တို႔ေၾကာင့္ျဖစ္ေနေသာ electric potential သည္ V₁, V₂, V₃, ................. စသည္တို႔အသိီးသီးျဖစ္ၾကၿပီး၊ စုစုေပါင္း electric potential V သည္
V = V₁ + V₂ + V₃ + ......................... ျဖစ္သည္။
Wednesday, February 8, 2017
ELECTRIC FIELD AND ELECTRIC FIELD INTENSITY
ELECTRIC FIELD AND ELECTRIC FIELD INTENSITY
လွ်ပ္စစ္စက္ကြင္း ႏွင့္ လွ်ပ္စစ္စက္ကြင္းျပင္းအားWhen a positive charge q is brought close to another positive charge Q , which is placed at a point, the charge q is found to be acted upon by a repulsive force. The repulsive force becomes greater as q gets nearer to Q. That is, Q has a field surrounding it where electric forces due to it may act. In other words, Q produces or set up an electric field around it.
Positive Charge q တစ္ခုကို အမွတ္တစ္ေနရာေတြထားထားေသာ အျခားေသာ Positive charge Q တစ္ခုနားကို ယူေဆာင္တည္ရွိေစေသာအခါ ၎ charge q ကို တြန္းအား (repulsive force) တစ္ခုသက္ေရာက္ေနပါသည္။ Charge q သည္ Charge Q နားသို႔ ပိုပိုနီးလာေလ တြန္းကန္အားသည္လည္း ပိုပိုၾကီးလာေလျဖစ္သည္။ ဆိုလိုသည္မွာ Charge Q ေၾကာင့္ electric force တို႔ျဖစ္ေပၚေနျပီး ၎ Charge Q ပတ္၀န္းက်င္တြင္ electric field သည္ Q ကို ၀ိုင္းရံထားသည္။ တစ္နည္းဆိုေသာ္ Charge Q သည္ ၎ပတ္၀န္းက်င္တြင္ လွ်ပ္စစ္စက္ကြင္းကိုတည္ေဆာက္ျဖစ္ေပၚေနေစပါသည္။
Fig. 8.3 Electric filed around charged bodies
အထက္ပါပံုတြင္ Charge Q မွ ထုတ္လႊတ္လိုက္ေသာ electric field သည္ charge q ေပၚသို႔ Force ကိုတိုက္ရိုက္သက္ေရာက္ေစပါသည္။ charge q ကို ဖယ္ရွားလိုက္ေသာ္လည္း Charge Q ၏ လွ်ပ္စစ္စက္ကြင္းသည္ ဆက္လွ်က္တည္ရွိေနပါဦးမည္။ ထိုျပင္ charge q ကို charge q ၏အနီးနားပတ္၀န္းက်င္၏မည္သည့္ေနရာတြင္ ထားထားေစကာမူ charge Q ၏ လွ်ပ္စစ္စက္ကြင္းေၾကာင့္ျဖစ္ေပၚေနေသာ repulsive force (တြန္းအား) သည္ charge q ေပၚတြင္ဆက္လွ်က္သက္ေရာက္ေနပါဦးမည္။ အဘယ္ေၾကာင့္ဆိုေသာ္ Charge Q သည္ repulsive force ကို ကိုယ္တိုင္လုပ္ေဆာင္ေနေသာေၾကာင့္ျဖစ္ပါသည္။ Charge q သည္လည္း ပတ္၀န္းက်င္တြင္ လွ်ပ္စစ္စက္ကြင္းကိုထုတ္လႊတ္ေနသည္ဟုေျပာ၍ရႏိုင္ပါသည္။ ထို႕ေၾကာင္း electric field ဆိုမွာ electric forces မ်ားသက္ေရာက္ေနေသာ ေနရာအပုိင္းအျဖစ္ အဓိပၸါယ္သတ္မွတ္ႏိုင္ပါသည္။
Not just the positive charges but the negative charges as well are surrounded by electric fields.
Positive charges မ်ားသာမက Negative charges မ်ားကို electric field မ်ား၀န္းရံထားၾကသည္။
We may say, then, that any electric charge gives rise to an electric field in its vicinity.
မည္သည္ electric charge မဆို ၎တို႔ပတ္၀န္းက်င္တြင္ electric field ကို ထုတ္လႊတ္ျဖစ္ေပၚေနေစသည္ဟု ကြ်န္ေတာ္တိုေျပာႏိုင္ပါသည္။
In order to test whether an electric field exists at a certain point, a test charge must be placed at that point. If in electric force is exerted on the test charge, then we can say that an electric filed exists at the point under consideration. Generally, a unit positive charge is considered as a test charge.
သိက်ေသာအမွတ္တစ္ေနရာတြင္ electric field တည္ရွိသည္မတည္ရွိသည္ကို စမ္းသတ္ရန္အလိုငွာ စမ္းသပ္လွ်ပ္စစ္တစ္ခုကို ထိုအမွတ္ေနရာတြင္ထားရွိေစရပါမည္။ ၎ test charge ေပၚသို႔ electric force ကိုသက္ေရာက္ေစလွ်င္ ထိုအေျခအေန၌ ၎အမွတ္တြင္ electric field သက္ေရာက္ေနသည့္ဟူ ကြ်န္ေတာ္တို႕ေတြေျပာႏိုင္ပါသည္။ အမာ်းအားျဖင့္ 1 Unit Positive Charge ကိုသာ test charge တစ္ခုအျဖစ္မွတ္ယူၾကသည္။
The Electric field Intensity
We have seen that when an electric charge is placed in an electric filed a force is exerted on it. If the charge is moved from a point to another point in the field, the magnitude and direction of the force acting upon it will change. This means that the the magnitude and direction of the force acting upon the charge will change in accordance with the change in position of the charge. It is necessary to know the electric field intensity in order to specify an electric filed. The electric filed intensity is defined as follows.Electric field တစ္ခုအတြင္း electric charge တစ္ခုကိုထားထားေသာအခ်ိန္တြင္ force တစ္ခုသက္ေရာက္ေစေၾကာင္းကို ကြ်န္ေတာ္တို႔ေတြသိျမင္ခဲ့ၾကၿပီးျဖစ္သည္။ ထိုစက္ကြင္းအတြင္း charge ကို point တစ္ေနရာမွ အျခား point တစ္ေနရာသို႔ ေရြ႕ေျပာင္းလိုက္လွ်င္ ၎ charge ေပၚသို႔သက္ေရာက္ေနေသာ force magnitude (ပမာဏ) ႏွင့္ direction (ဦးတည္ဘက္) တို႔သည္လည္း ေျပာင္းလဲးသြားလိမ္မည္။ ဆိုလိုသည္မွာ - charge ေပၚသို႔သက္ေရာက္ေနေသာ force ၏ magnitude and direction တို႔သည္ ၎ charge ၏ position (တည္ေနရာ) ေျပာင္းလဲျခင္းႏွင့္အညီလိုက္၍ေျပာင္းလဲေနပါလိမ္မည္။ Electric field ကို တိတိက်က်သိေစရန္အလို႔ငွာ electric field intensity (လွ်ပ္စစ္စက္ကြင္းျပင္းအား) ကို သိရန္လိုအပ္ပါသည္။ electric field intensity ကို ေအာက္ပါအတိုင္း အဓိပၸါယ္မွတ္ယူသည္။
The electric field intensity at a point in an electric field is the electric force acting upon a unit positive charge placed at that point. The electric field intensity is a vector quantity. The electric field intensity is represented by E.
electric field တစ္ခုအတြင္း အမွတ္တစ္ေနရာ၌ရွိေသာ electric field intensity ဆိုသည္မွာ ထိုအမွတ္၌ထားထားေသာ အေပါင္းတစ္ယူနစ္ charge အေပၚသို႔သက္ေရာက္ေနေသာ electric force ျဖစ္သည္။ electric field intensity သည္ vector quantity အမ်ဳိးအစားတစ္ခုျဖစ္သည္။ electric field intensity ကို အဂၤလိပ္အကၡရာ E ျဖင့္ကိုယ္စားျပဳသည္။
In Fig. 8.3 the force on q exerted by Q is F.
အထက္ပါပံု Fig.8.3 တြင္ charge Q အားျဖင့္ charge q ေပၚသို႔သက္ေရာက္ေနေသာ force သည္ F ျဖစ္သည္။
The force exerted by Q on a unit positive charge is F/q.
အေပါင္းတစ္ယူနစ္ charge ေပၚသို႔ charge Q ေၾကာင့္သက္ေရာက္ေစေသာ force သည္ F/q ျဖစ္သည္။
Since the force acting upon a unit positive charge is the electric field intensity, the electric filed intensity E can be expressed as
တစ္ယူနစ္ positive charge ေပၚတြင္သက္ေရာက္ေနေသာအားသည္ electric field intensity ျဖစ္ေသာေၾကာင့္ ၎ electric field intensity E ကို ေအာက္ပါ equation ျဖင့္ ေဖာ္ျပတြက္ခ်က္ႏိုင္ပါသည္။
အထက္ပါ equation မွ electric field intensity (E) ၏ ဦးတည္ဘက္သည္ Force ၏ ဦးတည္အတိုင္းျဖစ္သည္ကို ကြ်န္ေတာ္တိုေတြ႕ျမင့္ၾကရသည္။
In the SI system the unit of electric force F is newton (N) and the unit of electric charge q is coulomb (C). Thus, the unit of electric field intensity E is newton per coulomb (N C⁻¹).
SI system တြက္ခ်က္ရာတြင္ Force ၏ unit သည္ newton (N) ျဖစ္၍ electric charge q ၏ unit သည္ coulomb (C) ျဖင့္ တြက္ခ်က္ရသည္။ ထို႕ေၾကာင္ electric field intensity E ၏ ယူနစ္သည္ newton per coulomb (N C⁻¹) ျဖစ္သည္။
Equation can be rewritten as
တြက္ခ်က္မူ equation ကို ေအာက္ပါအတိုင္း ေရးသားႏိုင္သည္။
F = qE
If the values of q and E are known the force F acting upon q can be calculated from the about equation.
Charge q ႏွင္ electric field intensity တို႔၏ တန္ဖိုးကိုသိရွိထားလွ်င္ charge q ေပၚတြင္ သက္ေရာကေနေသာ force F တန္ဖိုးကို အထက္ပါ equation မွတြက္ခ်က္ယူႏိုင္သည္။
Calculation of the Electric Field Intensity from Coulomb's Law
The electric filed intensity at a point, a certain distance from the charge, can be calculated by using Coulomb's law.
၎ charge မွ သိက်ေသာအကြာအေ၀းရွိ အမွတ္တစ္ေနရာ၌ရွိေသာ electric field intensity ကို Coulomb's law ကို အသံုးျပဳၿပီး တြက္ယူႏုိင္သည္။
Fig. 8.4 Force on a charge in an electric field
We shall consider the electric field surrounding the charge Q, shown in Fig. 8.4 and then find the electric field intensity at a point A in the field, at a distance r from Q . Suppose that a small positive charge q is placed at A.
By Coulomb's law the force F on q due to Q is
The force on a unit positive charge due to Q is by definition the electric field intensity; thus
Charge Q ေၾကာင့္ တစ္ယူနစ္ positive charge (+1 C) ေပၚတြင္သက္ေရာက္ေသာအားသည္ electric field intensity ျဖစ္သည္။
Equation gives that the magnitude of the electric field intensity at the point A.
အထက္ပါ equation သည္ အမွတ္ A ေနရာတြင္တည္ရွိေနေသာ electric field intensity ၏ ပမာဏ (magnitude) ကိုတြက္ခ်က္ေပးသည္။
In the SI system the unit of the charge Q is coulomb (C) and the unit of distance r is metre (m). When these units and the SI unit of 1/4𝛑𝛆₀ are used in equation, the unit of E is newton per coulomb (N C⁻¹)
SI system တြင္ charge Q ၏ unit သည္ coulomb (C) ျဖစ္ၿပီး၊ အကြာအေ၀း distance r ၏ unit သည္ metre (m) ျဖစ္သည္။ အထက္ပါ equation တြင္ charge Q ႏွင့္ distance r တို႔၏ unit မ်ားႏ်ွင့္ 1/4𝛑𝛆₀ တို႔၏ unit မ်ားကို အသံုးျပဳလိုက္ေသာအခါ electric field intensity E ၏ unit သည္ newton per coulomb (N C⁻¹) အျဖစ္ရရွိသည္။
The direction of the electric filed intensity at the point A is along the line joining q and Q and away from Q.
အမွတ္ A ၌ ရွိေသာ electric field intensity ၏ ဦးတည္ဘက္သည္ charge q ႏွင့္ charge Q တို႔ ဆက္သြားထားေသာ မွ်ဥ္းလႈိင္းအတိုင္းသာျဖစ္ျပီး charge Q ၏ အေ၀းသို႔သာ ဦးတည္းသည္။ (မွတ္ခ်က္ q₁ ကို Q ဟုမွတ္ယူသည္။)
If a negative charge Q is put in place of the positive charge Q, the magnitude of the electric field intensity at the point A will not change, But its directions will be along the line joining q and Q and toward Q.
positive charge Q ေနရာတြင္ negative charge Q ကိုအစားထုိးလွ်င္ အမွတ္ A ၌ ရွိေသာ electric field intensity E ၏ magnitude ပမာဏသည္ မေျပာင္းပဲ၊ ၎ electric field intensity E ၏ ဦးတည္ဘက္သည္ charge q ႏွင့္ Charge Q ကိုဆက္သြယ္ေသာ line တစ္ေလွ်ာက္သာျဖစ္ျပီး charge Q ဆီသို႔ဦးတည္သြားမည္ျဖစ္သည္။
If the resultant electric field intensity at a point due to two or more charges to be found, the vector sum of electric field intensities at that point must be taken. This means that if the electric field intensities at a point due to the charges are E₁, E₂, E₃ ........... , then the resultant electric filed intensity E at that points is
အမွတ္တစ္ေနရာ၌ရွိေသာ electric field intensity သည္ charge ႏွစ္ခု သို႔မဟုတ္ မ်ားစြာေသာ charge မ်ားေၾကာင့္ျဖစ္ေပၚေနလွ်င္ ထိုအမွတ္၌ရွိေသာ electric field intensity ၏ vector ေပါင္းလဒ္ကို တြက္ယူရမည္ျဖစ္သည္။ ဆိုလိုသည္မွာ အမွတ္တစ္ေနရာ၌ရွိေသာ electric field intensity သည္ E₁, E₂, E₃ ........... , တို႔ျဖစ္ေနၾကလွ်င့္ ထိုအမွတ္၌ရွိေသာ အသာတင္ electric field intensity တန္ဖိုးသည္ ေအာက္ပါ vector sum အတိုင္းျဖစ္သည္။
E = E₁ + E₂ + E₃ + ..................
Tuesday, February 7, 2017
Example (2) in Chapter (8)
Example (2)
(a) Calculate the values of two equal charges if they repel one another with force of 0.1 N when situated 50 cm apart in vacuum.
(b) Calculate the values of two equal charges if they repel one another with a force of 0.1 N when situated 50 cm apart in a liquid whose permittivity is 10 times that of vacuum.
တူညီေသာ Charge ႏွစ္ခုကို ေလဟာနယ္ထဲတြင္ 50 cm ကြာ၍ထားထားၿပီး Force 0.1 N အားသက္ေရာက္ေနေသာ္ ၎ Charge ႏွစ္ခု၏တန္ဖိုးကို တြက္ျပပါ။
တူညီေသာ Charge ႏွစ္ခုကို vacuum ၏ permittivity ထက္ ၁၀ ဆ ႀကီးေသာ အရည္ထဲတြင္ 50 cm ကြာ၍ထားထားၿပီး အား 0.1 N သက္ေရာက္ေစလွ်င္ ၎ Charge ႏွစ္ခု၏တန္ဖိုးကိုတြက္ျပပါ။
Example (3) သို႔
(a) Calculate the values of two equal charges if they repel one another with force of 0.1 N when situated 50 cm apart in vacuum.
(b) Calculate the values of two equal charges if they repel one another with a force of 0.1 N when situated 50 cm apart in a liquid whose permittivity is 10 times that of vacuum.
တူညီေသာ Charge ႏွစ္ခုကို ေလဟာနယ္ထဲတြင္ 50 cm ကြာ၍ထားထားၿပီး Force 0.1 N အားသက္ေရာက္ေနေသာ္ ၎ Charge ႏွစ္ခု၏တန္ဖိုးကို တြက္ျပပါ။
တူညီေသာ Charge ႏွစ္ခုကို vacuum ၏ permittivity ထက္ ၁၀ ဆ ႀကီးေသာ အရည္ထဲတြင္ 50 cm ကြာ၍ထားထားၿပီး အား 0.1 N သက္ေရာက္ေစလွ်င္ ၎ Charge ႏွစ္ခု၏တန္ဖိုးကိုတြက္ျပပါ။
Example (3) သို႔
Exmaple (1) in Chapter (8)
Example (1)
Find the force between two charges of 1 C each that are 1 m apart.
အကြာအေ၀း 1 m ျခားထားေသာ 1 C ဆီအသီးသီးရွိၾကေသာ Charge ႏွစ္ခုၾကားတြင္ရွိေသာ Force ကိုရွာပါ။
Example (2) သို႔
Friday, February 3, 2017
Chapter 8
THE ELECTRIC FIELD (လွ်ပ္စစ္စက္ကြင္း)
Two electric charges, which are not in contact, can exert electrical forces on each other. The concept of electric field is used to explain this phenomenon.
ထိစပ္ေနျခင္းမရွိေသာ္လည္း လွ်ပ္စစ္ဓါတ္ႏွစ္ခုၾကားတြင္ တစ္ခုကိုတစ္ခု လွ်ပ္စစ္အားမ်ားသက္ေရာက္ေစႏိုင္ပါသည္။ ဒီျဖစ္စဥ္ကို ရွင္းလင္းတင္ျပရန္အတြက္ လွ်ပ္စစ္စက္ကြင္း၏အေဘာတရားမ်ားကို အသံုးျပဳခဲ့ပါသည္။
Just as there is a gravitational force between two masses so there is an electric force between two charged particles. Electrical forces bind electrons and nuclei to form atoms. In addition, these forces hold atoms to form molecules, liquids and solids. It has already been mentioned in mechanics that there are only four fundamental forces, namely, gravitational force, weak interaction, electromagnetic force and nuclear force. Of these forces gravitational and electromagnetic forces are long-range forces.
အရာ၀တၳဳႏွစ္ခုၾကားတြင္ ကမၻာေျမဆဲြအားသက္ေရာက္ေနသကဲ့သို႔ လွ်ပ္စစ္ဓါတ္ေဆာင္အမႈန္ႏွစ္ခုၾကားတြင္လည္း electric force (လွ်ပ္စစ္သက္ေရာက္အား) တစ္ခုသက္ေရာက္ေနပါသည္။ ၎ electric forces မ်ားသည္ atom အမႈန္တစ္ခုျဖစ္လာရန္ electrons မ်ားႏွင့္ nuclei မ်ားကို မကဲြကြာေအာင္တြဲေႏွာင္ထားပါသည္။ ထိုျပင္ ၎ electric forces မ်ားသည္ molecules မ်ား၊ အရည္မ်ား၊ အစိုင္အခဲမ်ားျဖစ္လာေအာင္ atom မ်ားကို ေပါင္းစည္းေစသည္။ အမည္အားျဖင့္ gravitational force (ကမၻာ႕ေျမဆြဲအား)၊ weak interaction (သတၱိၾကြအား)၊ electromagnetic force (လွ်ပ္စစ္သံလိုက္စက္ကြင္းအား)၊ nuclear force (နယူကလိယားအႏုျမဴအား) ဟူ၍ အေျခခံအားေလးမ်ဳိးရွိသည္ကို mechanics သင္ခန္းစာအခန္းမ်ားတြင္တင္ျပေပးခဲျပီးျဖစ္သည္။ ထိုအားေလးမ်ဳိးတြင္ gravitational force ႏွင့္ electromagnetic force ႏွစ္ခုသည္ တာေ၀သို႔တိုင္ေအာင္သက္ေရာက္ေစႏိုင္ေသာေၾကာင့္ ၎အားႏွစ္မ်ဳိးကို long-range forces မ်ားဟုေခၚသည္။
The French scientist, Coulomb, studied systematically the attractive and repulsive forces acting between pairs of charges and discovered a certain law. This law is called Coulomb's law and it states that:
ျပင္သစ္သိပၸံပညာရွင္ျဖစ္ေသာ Coulomb ဆိုသူသည္ လွ်ပ္စစ္ဓါတ္ႏွစ္ခုၾကားတြင္ သက္ေရာက္ေနေသာ ဆဲြအားႏွင့္တြန္းအားမ်ားကို နည္းစနစ္က်က်ေလ့လာခဲ့ျပီး၊ သိက်ေသခ်ာေသာနိယာမတစ္ခုကိုရွာေဖြေဖာ္ထုတ္ခဲ့သည္။ ၎နိယာမကို Coulomb's Law ဟုေခၚဆိုျပီး ေဖာ္ျပခ်က္မွာေအာက္ပါအတိိုင္းျဖစ္သည္။
လွ်ပ္စစ္ဓါတ္ႏွစ္ခုၾကားတြင္ သက္ေရာက္ေနေသာသက္ေရာက္အားသည္ ၎လွ်ပ္စစ္ဓါတ္ပမာဏမ်ားေျမာက္လဒ္ျဖင့္ တိုက္ရိုက္အခ်ဳိးက်ျပီး၊ ၎လွ်ပ္စစ္ဓါတ္ႏွစ္ခု၏အကြာေ၀းႏွင့္ေျပာင္းျပန္အခ်ိဳးက်သည္။ ဆိုလိုသည္မွာ Chargeႏွစ္ခု ပမာဏမ်ားလာလွ်င္ Force မ်ားလာျပီး၊ Charge ႏွစ္ခုပိုေ၀းေလေလ Force သည္လည္း နည္းလာေလေလျဖစ္သည္။
အထက္ပါပံုတြင္ q₁ ႏွင့္ q₂ တို႔သည္ electric charges မ်ားျဖစ္ျပီး r သည္ ၎ charges ႏွစ္ခုၾကားအကြားအေ၀းျဖစ္ေသာ္ သခ်ာၤနည္းျဖင့္ Coulomb's law ကို ေအာက္ပါအတိုင္းတင္ျပႏိုင္သည္။
Since the force F is inversely proportional to r², Coulomb's law is also called an inverse square law.
သက္ေရာက္အား F သည္ အကြာအေ၀း r ႏွစ္ထပ္ကိန္းႏွင့္ ေျပာင္းျပန္အခ်ိဳးက်ေနေသာေၾကာင့္ Coulomb's law ကို ႏွစ္ထပ္ကိန္းေျပာင္းျပန္နိယာမ inverse square law ဟုလည္းထပ္မံေခၚဆုိပါသည္။
In equation, K is a constant. The value of K depends upon the units of F, q₁, q₂ and r and upon the medium in which the charge q₁ and q₂ are located.
အထက္ပါ equation တြင္ K တန္ဖိုးသည္ ကိန္းေသျဖစ္သည္။ K ၏တန္ဖိုးသည္ F, q₁, q₂, r တို႔ႏွင့္ q₁, q₂ တို႔တည္ရွိေသာၾကားခံအရာ၀တၳဳ (ၾကားခံနယ္) အေပၚတြင္သာ မီွတည္ေျပာင္းလဲပါလိမ္မည္။
Electrical force is, of course, a vector quantity. Equation only gives the magnitude of the force between two electric charges. The direction of electrical force is always along the ling joining the two charges. If the charges are like charges the force between them is repulsive and is directed outward. If the charges is unlike charges the force between them is attractive and directed inward.
အမွန္တကယ္တြင္ electrical force သည္ vector quantity သာျဖစ္သည္။ အထက္ပါ equation သည္ လွ်ပ္စစ္ဓါတ္ႏွစ္ခုၾကားတြင္ျဖစ္ေပၚေနေသာ force ၏ပမာဏတန္ဖိုးကိုသာေဖၚျပေပးပါသည္။ electrical force ၏ ဦးတည္ဘက္သြားရာသည္ ၎ charges ႏွစ္ခုကိုဆက္သြယ္ထားေသာ မ်ဥ္းေၾကာင္းလႈိင္းအတိုင္းသာ ဦးတည္ေနၾကသည္။ Charges မ်ား အမ်ဳိးအစားတူေနလွ်င္ Force သည္ တြန္းကန္အားျဖစ္ၿပီး၊ ဦးတည္ဘက္သြားရာသည္ အျပင္သို႔ျဖစ္သည္။ Charges မ်ား အမ်ိဳးအစားမတူၾကလွ်င္ Force သည္ ဆဲြအားျဖစ္ျပီး၊ ဦးတည္ဘက္သြားရာသည္ အတြင္းသို႔ျဖစ္သည္ကို ေအာက္ပါပံုတြင္ၾကည့္ပါ။
In the SI system, charge q is measured in coulomb, the distance r in meter and the force F in newton. In The SI system,
SI စနစ္တြင္၊ Charge q ကို coulomb (C) unit ျဖင့္တိုင္းတားတြက္ခ်က္ၿပီး၊ အကြာအေ၀း r ကို meter (m) ျဖင့္လည္းေကာင္း the force F ကို newton (N) ယူနစ္တို႔ျဖင့္လည္းေကာင္း တိုင္းတာတြက္ခ်က္ၾကသည္။ SI စနစ္တြင္ K တန္ဖုိး equation မွာ
Where 𝞮 is a constant called the permittivity of the medium in which the charges are located. Then, equation can be rewritten as
𝞮 ကို charges မ်ားတည္ရွိေသာ ၾကားခံနယ္၏ျဖတ္သန္းႏႈန္းကိန္းေသျဖစ္ေနေစသာအခါ K တန္ဖိုး equation မွာ
Where charges are located in Vacuum,
Charges မ်ား ေလဟာနယ္ (Vacuum) အတြင္းတည္ရွိေနေသာအခါ
Therefore,
where Ɛ₀ is the permittivity of vacuum and
Ɛ₀ ကို ေလဟာနယ္ျဖတ္သန္းႏႈန္းမွတ္ယူေသာအခါ Ɛ₀ ၏ တန္ဖိုးမွာ
and the value of K in vacuum is
ထိုေၾကာင့္ ေလဟာနယ္ထဲတြင္ရွိေသာ K ၏တန္ဖိုးမွာ
However, for convenience in calculation, the value of K in vacuum will be taken as
သို႔ေသာ္လည္း အျပည္ျပည္ဆိုင္ရာသြက္ခ်က္မႈမ်ားတြင္ ေလဟာနယ္တြင္းရွိ K ၏တန္ဖိုးကို ေအာက္ပါအတိုင္းတြက္ယူၾကသည္။
The value of K in air is approximately equal to that of K in vacuum.
ေလထဲတြင္ရွိေသာ K ၏ တန္ဖိုးႏွင့္ ေလဟာနယ္ထဲတြင္ရွိေသာ K ၏တန္ဖိုးတို႔သည္ ထပ္တူနီးပါ တန္ဖိုးညီေနၾကသည္။
Two electric charges, which are not in contact, can exert electrical forces on each other. The concept of electric field is used to explain this phenomenon.
ထိစပ္ေနျခင္းမရွိေသာ္လည္း လွ်ပ္စစ္ဓါတ္ႏွစ္ခုၾကားတြင္ တစ္ခုကိုတစ္ခု လွ်ပ္စစ္အားမ်ားသက္ေရာက္ေစႏိုင္ပါသည္။ ဒီျဖစ္စဥ္ကို ရွင္းလင္းတင္ျပရန္အတြက္ လွ်ပ္စစ္စက္ကြင္း၏အေဘာတရားမ်ားကို အသံုးျပဳခဲ့ပါသည္။
COULOMB'S LAW
Just as there is a gravitational force between two masses so there is an electric force between two charged particles. Electrical forces bind electrons and nuclei to form atoms. In addition, these forces hold atoms to form molecules, liquids and solids. It has already been mentioned in mechanics that there are only four fundamental forces, namely, gravitational force, weak interaction, electromagnetic force and nuclear force. Of these forces gravitational and electromagnetic forces are long-range forces.
အရာ၀တၳဳႏွစ္ခုၾကားတြင္ ကမၻာေျမဆဲြအားသက္ေရာက္ေနသကဲ့သို႔ လွ်ပ္စစ္ဓါတ္ေဆာင္အမႈန္ႏွစ္ခုၾကားတြင္လည္း electric force (လွ်ပ္စစ္သက္ေရာက္အား) တစ္ခုသက္ေရာက္ေနပါသည္။ ၎ electric forces မ်ားသည္ atom အမႈန္တစ္ခုျဖစ္လာရန္ electrons မ်ားႏွင့္ nuclei မ်ားကို မကဲြကြာေအာင္တြဲေႏွာင္ထားပါသည္။ ထိုျပင္ ၎ electric forces မ်ားသည္ molecules မ်ား၊ အရည္မ်ား၊ အစိုင္အခဲမ်ားျဖစ္လာေအာင္ atom မ်ားကို ေပါင္းစည္းေစသည္။ အမည္အားျဖင့္ gravitational force (ကမၻာ႕ေျမဆြဲအား)၊ weak interaction (သတၱိၾကြအား)၊ electromagnetic force (လွ်ပ္စစ္သံလိုက္စက္ကြင္းအား)၊ nuclear force (နယူကလိယားအႏုျမဴအား) ဟူ၍ အေျခခံအားေလးမ်ဳိးရွိသည္ကို mechanics သင္ခန္းစာအခန္းမ်ားတြင္တင္ျပေပးခဲျပီးျဖစ္သည္။ ထိုအားေလးမ်ဳိးတြင္ gravitational force ႏွင့္ electromagnetic force ႏွစ္ခုသည္ တာေ၀သို႔တိုင္ေအာင္သက္ေရာက္ေစႏိုင္ေသာေၾကာင့္ ၎အားႏွစ္မ်ဳိးကို long-range forces မ်ားဟုေခၚသည္။
The French scientist, Coulomb, studied systematically the attractive and repulsive forces acting between pairs of charges and discovered a certain law. This law is called Coulomb's law and it states that:
The electric force between two charges is directly proportional to the product of the charges and inversely proportional to square of the distance between them.
ျပင္သစ္သိပၸံပညာရွင္ျဖစ္ေသာ Coulomb ဆိုသူသည္ လွ်ပ္စစ္ဓါတ္ႏွစ္ခုၾကားတြင္ သက္ေရာက္ေနေသာ ဆဲြအားႏွင့္တြန္းအားမ်ားကို နည္းစနစ္က်က်ေလ့လာခဲ့ျပီး၊ သိက်ေသခ်ာေသာနိယာမတစ္ခုကိုရွာေဖြေဖာ္ထုတ္ခဲ့သည္။ ၎နိယာမကို Coulomb's Law ဟုေခၚဆိုျပီး ေဖာ္ျပခ်က္မွာေအာက္ပါအတိိုင္းျဖစ္သည္။
လွ်ပ္စစ္ဓါတ္ႏွစ္ခုၾကားတြင္ သက္ေရာက္ေနေသာသက္ေရာက္အားသည္ ၎လွ်ပ္စစ္ဓါတ္ပမာဏမ်ားေျမာက္လဒ္ျဖင့္ တိုက္ရိုက္အခ်ဳိးက်ျပီး၊ ၎လွ်ပ္စစ္ဓါတ္ႏွစ္ခု၏အကြာေ၀းႏွင့္ေျပာင္းျပန္အခ်ိဳးက်သည္။ ဆိုလိုသည္မွာ Chargeႏွစ္ခု ပမာဏမ်ားလာလွ်င္ Force မ်ားလာျပီး၊ Charge ႏွစ္ခုပိုေ၀းေလေလ Force သည္လည္း နည္းလာေလေလျဖစ္သည္။
Since the force F is inversely proportional to r², Coulomb's law is also called an inverse square law.
သက္ေရာက္အား F သည္ အကြာအေ၀း r ႏွစ္ထပ္ကိန္းႏွင့္ ေျပာင္းျပန္အခ်ိဳးက်ေနေသာေၾကာင့္ Coulomb's law ကို ႏွစ္ထပ္ကိန္းေျပာင္းျပန္နိယာမ inverse square law ဟုလည္းထပ္မံေခၚဆုိပါသည္။
In equation, K is a constant. The value of K depends upon the units of F, q₁, q₂ and r and upon the medium in which the charge q₁ and q₂ are located.
အထက္ပါ equation တြင္ K တန္ဖိုးသည္ ကိန္းေသျဖစ္သည္။ K ၏တန္ဖိုးသည္ F, q₁, q₂, r တို႔ႏွင့္ q₁, q₂ တို႔တည္ရွိေသာၾကားခံအရာ၀တၳဳ (ၾကားခံနယ္) အေပၚတြင္သာ မီွတည္ေျပာင္းလဲပါလိမ္မည္။
Electrical force is, of course, a vector quantity. Equation only gives the magnitude of the force between two electric charges. The direction of electrical force is always along the ling joining the two charges. If the charges are like charges the force between them is repulsive and is directed outward. If the charges is unlike charges the force between them is attractive and directed inward.
အမွန္တကယ္တြင္ electrical force သည္ vector quantity သာျဖစ္သည္။ အထက္ပါ equation သည္ လွ်ပ္စစ္ဓါတ္ႏွစ္ခုၾကားတြင္ျဖစ္ေပၚေနေသာ force ၏ပမာဏတန္ဖိုးကိုသာေဖၚျပေပးပါသည္။ electrical force ၏ ဦးတည္ဘက္သြားရာသည္ ၎ charges ႏွစ္ခုကိုဆက္သြယ္ထားေသာ မ်ဥ္းေၾကာင္းလႈိင္းအတိုင္းသာ ဦးတည္ေနၾကသည္။ Charges မ်ား အမ်ဳိးအစားတူေနလွ်င္ Force သည္ တြန္းကန္အားျဖစ္ၿပီး၊ ဦးတည္ဘက္သြားရာသည္ အျပင္သို႔ျဖစ္သည္။ Charges မ်ား အမ်ိဳးအစားမတူၾကလွ်င္ Force သည္ ဆဲြအားျဖစ္ျပီး၊ ဦးတည္ဘက္သြားရာသည္ အတြင္းသို႔ျဖစ္သည္ကို ေအာက္ပါပံုတြင္ၾကည့္ပါ။
In the SI system, charge q is measured in coulomb, the distance r in meter and the force F in newton. In The SI system,
SI စနစ္တြင္၊ Charge q ကို coulomb (C) unit ျဖင့္တိုင္းတားတြက္ခ်က္ၿပီး၊ အကြာအေ၀း r ကို meter (m) ျဖင့္လည္းေကာင္း the force F ကို newton (N) ယူနစ္တို႔ျဖင့္လည္းေကာင္း တိုင္းတာတြက္ခ်က္ၾကသည္။ SI စနစ္တြင္ K တန္ဖုိး equation မွာ
Where 𝞮 is a constant called the permittivity of the medium in which the charges are located. Then, equation can be rewritten as
𝞮 ကို charges မ်ားတည္ရွိေသာ ၾကားခံနယ္၏ျဖတ္သန္းႏႈန္းကိန္းေသျဖစ္ေနေစသာအခါ K တန္ဖိုး equation မွာ
Where charges are located in Vacuum,
Charges မ်ား ေလဟာနယ္ (Vacuum) အတြင္းတည္ရွိေနေသာအခါ
where Ɛ₀ is the permittivity of vacuum and
Ɛ₀ ကို ေလဟာနယ္ျဖတ္သန္းႏႈန္းမွတ္ယူေသာအခါ Ɛ₀ ၏ တန္ဖိုးမွာ
and the value of K in vacuum is
ထိုေၾကာင့္ ေလဟာနယ္ထဲတြင္ရွိေသာ K ၏တန္ဖိုးမွာ
သို႔ေသာ္လည္း အျပည္ျပည္ဆိုင္ရာသြက္ခ်က္မႈမ်ားတြင္ ေလဟာနယ္တြင္းရွိ K ၏တန္ဖိုးကို ေအာက္ပါအတိုင္းတြက္ယူၾကသည္။
The value of K in air is approximately equal to that of K in vacuum.
ေလထဲတြင္ရွိေသာ K ၏ တန္ဖိုးႏွင့္ ေလဟာနယ္ထဲတြင္ရွိေသာ K ၏တန္ဖိုးတို႔သည္ ထပ္တူနီးပါ တန္ဖိုးညီေနၾကသည္။
